Dividing a Polynomial by a Binomial

We can multiply x - 2 and x + 5 to get (x - 2)(x + 5) = x² + 3x - 10.

So if we divide x² + 3x - 10 by the factor x - 2, we should get the other factor x + 5. This division is not done like division by a monomial; it is done like long division of whole numbers. We get the first term of the quotient by dividing the first term of x - 2 into the first term of x² + 3x - 10. Divide x² by x to get x.

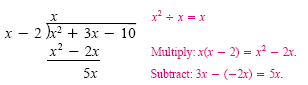
Now bring down -10. We get the second term of the quotient (below) by dividing the first term of x - 2 into the first term of 5x - 10. Divide 5x by x to get 5.

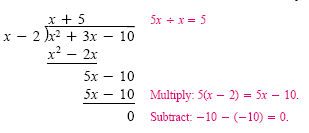
So the quotient is x + 5 and the remainder is 0. If the remainder is not 0, then

dividend = (divisor)(quotient) + (remainder).

If we divide each side of this equation by the divisor, we get

When dividing polynomials, we must write the terms of the divisor and the dividend in descending order of the exponents. If any terms are missing, as in the next example, we insert terms with a coefficient of 0 as placeholders. When dividing polynomials, we stop the process when the degree of the remainder is smaller than the degree of the divisor.

 

Example 1

Dividing polynomials

Find the quotient and remainder for (3x⁴ - 2 - 5x) ÷ (x² - 3x).

Solution

Rearrange 3x⁴ - 2 - 5x as 3x⁴ - 5x - 2 and insert the terms 0x³ and 0x²:

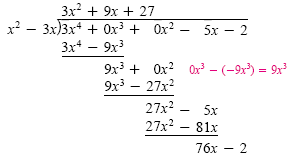
Helpful hint

Students usually have the most difficulty with the subtraction part of long division. So pay particular attention to that step and double check your work.

The quotient is 3x² + 9x + 27, and the remainder is 76x - 2. Note that the degree of the remainder is 1, and the degree of the divisor is 2. To check, verify that (x² - 3x)(3x² + 9x + 27) + 76x - 2 = 3x⁴ - 5x - 2.

 

Example 2

Rewriting a ratio of two polynomials

Write 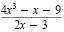in the form quotient

Solution

Divide 4x³ - x - 9 by 2x 3. Insert 0 · x² for the missing term.

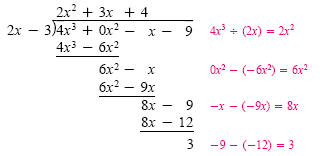
Since the quotient is 2x² + 3x + 4 and the remainder is 3, we have

To check the answer, we must verify that (2x - 3)(2x² + 3x + 4) + 3 = 4x³ - x - 9.