Solving Equations with Variables on Each Side
After studying this lesson, you will be able to:
- Solve equations with variables on each side of the equal
sign.
- Solve equations with parentheses and other grouping
symbols.
Steps for Solving Equations with Variables on Each
Side and with Parentheses
1. Remove parentheses by multiplying
2. Collect like terms on each side of the equal sign
3. Get the variables together on one side of the equation and
get the numbers together on the other side of the equation.
4. Isolate the variable by "undoing" the operation
(do this until the variable is by itself)
- "undo" addition and subtraction first
- next, "undo" multiplication and division
5. Check by substituting the solution into the original
equation
Example 1
| 3(x + 4) - 5 = 2x - 2 |
This equation has parentheses, so we have
to remove them first and we do so by multiplying (using
the distributive property). |
| 3x + 12 - 5 = 2x - 2 |
After distributing, we no longer have
parentheses, but we do have like terms on the left side
that need to be collected (12 -5 ) |
| 3x + 7 = 2x - 2 |
Collecting the like terms gives us this
equation |
| 3x + 7 - 2x = 2x - 2 - 2x |
First, we need to get the variables
together. It doesn't matter if we put them on the left
side or the right side. Let's put them together on the
left side this time. To do that, we move 2x to the other
side by subtracting 2x from each side. Notice that we
line up the like terms. (-2x is lined up with 3x so that
it is easier to deal with.) |
| x + 7 = - 2 |
After collecting like terms (3x -2x) we
now have an equation where the variables are now
together. Now, we work this as a 2-step equation. |
| x + 7 - 7 = - 2 - 7 |
We need to "undo" +7, so we
subtract 7 from each side. |
| x = -9 |
This gives us the solution |
Check:
substitute -9 for each x in the original equation
3 ( -9 + 4 ) - 5 = 2 (-9 ) -2
3 ( -5 ) -5 = 2 (-9 ) -2 Adding the -9 + 4
-15 - 5 = -18 - 2 Do the multiplication
-20 = -20
Example 2
| 5 + 2(x + 4) = 5(x - 3) + 10 |
This equation has 2 sets of parentheses,
so we have to remove them first and we do so by
multiplying (using the distributive property). |
| 5 + 2x + 8 = 5x - 15 + 10 |
After distributing, we no longer have
parentheses, but we do have like terms on the each side
that need to be collected (5 + 8 on the left and -15 + 10
on the right ) |
| 2x + 13 = 5x - 5 |
Collecting the like terms gives us this
equation |
| 2x + 13 - 2x = 5x - 5 - 2x |
First, we need to get the variables
together. It doesn't matter if we put them on the left
side or the right side. Let's put them together on the
right side this time. To do that, we move 2x to the other
side by subtracting 2x from each side. Notice that we
line up the like terms. (-2x is lined up with 5x so that
it is easier to deal with.) |
| 13 = 3x - 5 |
After collecting like terms (5x - 2x) we
now have an equation where the variables are now
together. Now, we work this as a 2-step equation. |
| 13 + 5 = 3x - 5 + 5 |
We need to "undo" -5, so we add
5 to each side. This gives us 18 = 3x
|
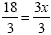 |
We need to "undo" 3 times x so
we divide both sides by 3 |
| 6 = x |
This gives us the solution |
Check:
substitute 6 for each x in the original equation
5 + 2 ( 6 + 4 ) = 5 ( 6 - 3 ) + 10
5 + 2 (10) = 5 (3) +10 doing the parentheses first
5 + 20 = 15 + 10 doing the multiplication
25 = 25 doing the addition
|