Solving Absolute Value Inequalities
Solving an Absolute Value Inequality of the Form
| x| < a
Example 1
Solve: -6|4x| > -72
| Solution
Step 1 Isolate the absolute value.
Divide both sides by -6 and reverse
the direction of the inequality symbol.
Simplify.
Step 2 Make the substitution w = 4x.
Step 3 Use the Absolute Value
Principle to solve for w.
Step 4 Replace w with 4x.
Replace w with 4x.
Step 5 Solve for x.
Divide all three parts by 4. |
-6|4x| > -72

|4x| < 12
|w| < 12
-12 < w < 12
-12 < 4x < 12
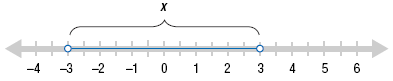
-3 < x < 3 |
Notes:
1) When multiplying or dividing both sides of
an inequality by a negative number,
reverse the direction of the inequality.
2) The compound inequality -3 < x < 3 is
read “-3 is less than x†and “x is less
than 3â€.
Step 6 Check the answer.
We leave the check to you.
So, the solution is -3 < x
< 3.
Example 2
Solve: -4 + 5|2x
- 4| < 36
| Solution
Step 1 Isolate the absolute value.
Add 4 to both sides.
Divide both sides by 5.
Step 2 Make the substitution w = 2x
- 4.
Step 3 Use the Absolute Value
Principle to solve for w.
Step 4 Replace w with 2x - 4.
Step 5 Solve for x.
Add 4 to all three parts.
Divide each part by 2. |
-4 + 5|2x
- 4| < 36
5|2x - 4| < 40
|2x - 4| < 8
|w| < 8
-8 < w <
8
-8 < 2x -
4 < 8
4 < 2x < 12
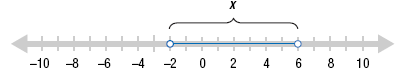
-2 < x <
6 |
Step 6 Check the answer.
We leave the check to you.
So, the solution is -2 < x
< 6.
Note:
-8 < 2x - 4
< 8 is a compound inequality since it contains
two inequality symbols.
A compound inequality is solved when the
variable has been isolated in the middle
part.
For example, -2 < x
< 6 is solved.
Remember, absolute value always represents a nonnegative number.
Therefore, some absolute value inequalities have no solution, as the next
example shows.
Example 3
Solve: |x| + 6 < 4
| Solution
Step 1 Isolate the absolute value.
Subtract 6 from both sides. |
|x| + 6 < 4
|x| < -2 |
For any value of x, we know that |x| is a nonnegative number. Thus,
|x| cannot be less than -2.
Therefore, |x| + 6 < 4 has no solution.
|