Solving Quadratic Equations by Completing the
Square
Example Solve by completing the square: 3x2 - 30 = -21x
Solution
| Step 1 Isolate the x2-term and the x-term
on one side of the equation.
Add 21x to both sides.
Add 30 to both sides. |
3x2 - 30 =
-21x
3x2 + 21x - 30 = 0
3x2 + 21x = 30 |
| Step 2 If the coefficient of x2 is not 1,
divide both sides of the equation
by the coefficient of x2.
Divide both sides by 3. |
x2 + 7x = 10 |
Step 3 Find the number that completes
the square: Multiply the coefficient
of x by
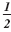 . Square the result. . Square the result.
The coefficient of the x-term is 7.
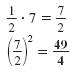 |
|
| Step 4 Add the result of Step 3 to both
sides of the equation.
Add
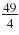 to both sides. to both sides.
Simplify the right side.
The result is
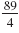 . . |

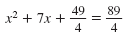 |
| Step 5 Write the trinomial as the
square of a binomial.
|
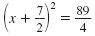 |
| Step 6 Finish solving using the
Square Root Property. Use the Square Root Property. |
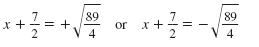 |
For each equation, subtract
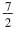 from both sides and simplify
the radical. from both sides and simplify
the radical. |
 |
| Step 7 Check each solution.
We leave the check for you. |
|
The solutions of 3x2 - 30 = -21x are
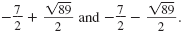
Note:
To divide both sides of an equation by 3,
we divide each term by 3, like this:
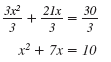
We can use the “plus or minus†symbol to
write the solutions like this
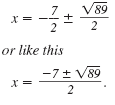
|